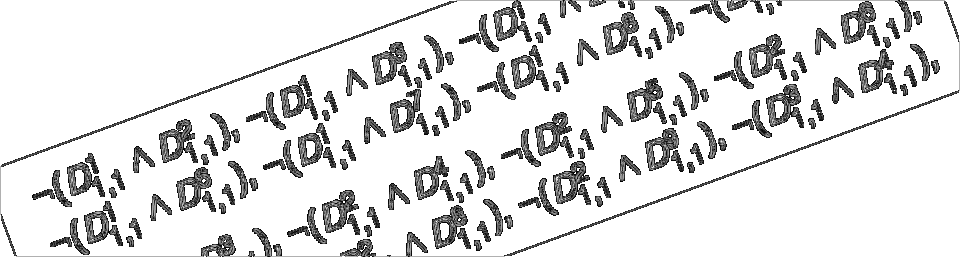@InProceedings{schmitt2017,
author = {Peter H. Schmitt},
editor = {Renate A. Schmidt and Cl{\'a}udia Nalon},
title = {A Mechanizable First-Order Theory of Ordinals},
booktitle = {26th International Conference on Automated
Reasoning with Analytic Tableaux and Related
Methods ({TABLEAUX} 2017)},
venue = {Bras{\'{\i}}lia, Brazil},
eventdate = {2017-09-25/2017-09-28},
year = {2017},
month = aug,
series = {LNCS},
volume = {10501},
publisher = {Springer},
doi = {10.1007/978-3-319-66902-1_20},
pages = {331--346},
abstract = {We present a first-order theory of ordinals
without resorting to set theory. The theory
is implemented in the {\KeY} program
verification system which is in turn used
to prove termination of a Java program
computing the Goodstein sequences.}
}
A Mechanizable First-Order Theory of Ordinals
| Autor(en): | Peter H. Schmitt |
|---|---|
| In: | 26th International Conference on Automated Reasoning with Analytic Tableaux and Related Methods (TABLEAUX 2017) |
| Verleger: | Springer |
| Reihe: | LNCS |
| Band: | 10501 |
| Jahr: | 2017 |
| Seiten: | 331-346 |
| DOI: | 10.1007/978-3-319-66902-1_20 |
Abstract
We present a first-order theory of ordinals without resorting to set theory. The theory is implemented in the KeY program verification system which is in turn used to prove termination of a Java program computing the Goodstein sequences.